Training and development of junior schoolchildren. Teaching primary schoolchildren at home. Development of mental functionsPerception

Slide 5

Speech and emotional communicationTypes of behavior in situations of frustration

Adequately loyal Apologizes if he was wrong, fearlessly but respectfully looks into the eyes of his opponent, Reaches this peak of adaptive behavior rarely, in individual situations favorable to himself. Inadequately loyal Hastens to apologize without analyzing the situation, submits to the opposing side, readiness to accept aggression crushes the child, dominates him. Adequately disloyal, aggressive “You’re a fool!” Open aggression in response to aggression puts the child in a position of equality; the struggle of ambitions will determine the winner through the ability to provide strong-willed resistance, without the use of physical force. Adequately disloyal, ignoring Openly ignoring in response to aggression can put the child above the situation. This position helps maintain self-esteem and a sense of personality. It is important to have sufficient intuition and reflection so as not to overdo it. Passive, inactive No communication occurs, the child avoids communication, withdraws (pulls his head into his shoulders, looks into a certain space in front of him, turns away, lowers his eyes, etc.). The situation is dangerous because the child may lose self-esteem and self-confidence .

Slide 6

Communication styles offered by adults in the family and at school

FAMILY Authoritarian style Liberal-permissive style Overprotective style Value style Alienated style SCHOOL Imperative (authoritarian) style Democratic style Liberal-permissive (anti-authoritarian).

Slide 7

Mental development Oral and written language

Slide 8

Correct speech

CORRECTNESS OF ORAL SPEECH Grammatical correctness; Orthoepic correctness; Pronunciation accuracy. CORRECTNESS OF WRITTEN SPEECH Grammatical (construction of sentences, formation of morphological forms); Spelling; Punctuation.

Slide 9

Development of mental functions Thinking

FEATURES OF THINKING DEVELOPMENT IN PRIMARY SCHOOL AGE Thinking becomes the dominant function; The transition from visual-figurative to verbal-logical thinking is completed; The emergence of logically correct reasoning; Use of specific operations; Formation of scientific concepts; Development of the foundations of conceptual (theoretical) thinking; The emergence of reflection; Manifestation of individual differences in types of thinking: theorists; practices; artists.

Slide 10

Development of mental functionsAttention

FEATURES OF ATTENTION DEVELOPMENT IN PRIMARY SCHOOL AGE Predominance of involuntary attention; Distractibility; Small attention span; Low attention span (junior schoolchildren 10-20 minutes, teenagers 40-45 minutes, high school students 45-50 minutes); It is difficult to switch and distribute attention; Development of voluntary attention; Individual attention options.

Slide 11

Development of mental functions Memory

FEATURES OF MEMORY DEVELOPMENT IN PRIMARY SCHOOL AGE: Developed mechanical memory; Development of semantic memory; Developed involuntary memory; Development of voluntary memory; Development of meaningful memorization; Ability to use mnemonics.

Slide 12

Development of mental functionsmemory

MNEMONIC TECHNIQUES FOR JUNIOR SCHOOLCHILDREN Dividing the text into semantic parts; coming up with titles for different parts; planning. Tracing the main lines of meaning; Isolation of semantic reference points or words; Returning to already read parts of the text to clarify their content; Mentally recalling the part read and reproducing all the material out loud or silently; Rational techniques for learning by heart. CONSEQUENCES OF THE APPLICATION OF MNEMONICS BY JUNIOR SCHOOLCHILDREN Understanding of educational material; Linking educational material with what has been completed; Inclusion in the general system of knowledge available to the child; Meaningful material is easily “extracted” from the system of connections and meanings; The educational material is much easier for the student to reproduce.

Slide 13

Development of mental functionsPerception

FEATURES OF PERCEPTION IN JUNIOR SCHOOLCHILDREN Perception at the beginning of the period is not sufficiently differentiated (6 and 9 are confused); Identification of the most striking properties of objects (color, shape, size); Observation skills develop; The emergence of synthesizing perception (analyzing in preschoolers);

Slide 14

Crisis 7th summer

Slide 15

General characteristics of educational activities

STRUCTURE OF LEARNING ACTIVITY (D.B. Elkonin): LEARNING TASK - what the student must learn, the method of action to be learned; LEARNING ACTIONS - what the student must do to form a model of an acquired action and reproduce this model; CONTROL ACTION – comparison of the reproduced action with a sample; ACTION OF ASSESSMENT - determination of how much the student has achieved the result, the degree of changes that have occurred in the child himself.

Slide 16

School readiness

PERSONAL READINESS FOR SCHOOL TRAINING the child’s desire for a new social position: initially the attractiveness of external attributes (briefcase, uniform, etc.); need for new social contacts. formation of a student’s internal position: the influence of close adults; the influence and attitude of other children; the opportunity to rise to a new age level in the eyes of younger ones; the opportunity to become equal in position with elders; attitude towards learning as a more significant activity than a preschooler’s play.

Slide 17

Personal readiness (continued) the formation of an extra-situational-personal form of communication with adults (according to M.I. Lisina): an adult is an indisputable authority, a role model; they are not offended by an adult’s comments, but rather try to correct mistakes; adequate understanding of the teacher’s position, his professional role; understanding the conventions of school communication, adequate submission to school rules. cooperative communication with peers prevails over competitive communication; the presence of a certain attitude towards oneself: the child’s adequate attitude towards his abilities, work results, behavior; a certain level of development of self-awareness; self-esteem should not be inflated and undifferentiated; motivational readiness to learn (cognitive need is stronger than the need for play (N.I. Gutkina’s method: listening to a fairy tale or playing with toys)); specific development of the sphere of voluntariness: the ability to fulfill the teacher’s educational requirements, given orally; work according to a visually perceived pattern; the ability to navigate a complex system of requirements (simultaneously following a model in one’s work and taking into account certain additional rules).

Slide 18

INTELLECTUAL READINESS FOR SCHOOL TRAINING Certain development of the level of thought processes: the ability to generalize, compare objects; classify, identify essential features; determine cause-and-effect relationships; ability to draw conclusions. The presence of a certain breadth of ideas: figurative ideas; spatial representations. Appropriate speech development; Cognitive activity.

Slide 19

TRAINING FROM 6 YEARS OLD

FEATURES OF 6-YEAR-OLD CHILDREN (from the point of view of schooling) thinking features corresponding to preschool age: predominance of involuntary memory; short duration of productive attention (10-15 minutes); predominance of visual-figurative thinking; cognitive motives adequate to learning tasks are unstable and situational; inflated self-esteem: lack of understanding of the criteria for pedagogical evaluation; a teacher’s assessment of their work is perceived as an assessment of their personality; A negative assessment does not cause a desire to redo it, but causes anxiety and a state of discomfort. general instability of behavior; dependence on emotional state; social instability; an urgent need for direct emotional contacts (in the formalized conditions of schooling this need is not satisfied); fast fatiguability; high distractibility;

Slide 20

Slide 21

Diagnosis of children from 6-7 to 10-11 years old

METHODOLOGICAL MATERIAL

Slide 22

Methodology L.Ya. Yasyukova

Purpose of the method Determination of readiness for school. Forecast and prevention of learning problems in primary school. The technique diagnoses: the speed of information processing, voluntary attention, short-term auditory and visual memory, speech development, conceptual and abstract thinking, characteristics of the prevailing emotional background, the energy balance of the child’s body and adaptive capabilities, personal learning potential (self-esteem, emotional attitudes towards school, family situation, etc.).

Slide 23

Cattell Factorial Personality Inventory (children) (from 7 to 12)

Purpose of the technique R. Cattell's factorial personality questionnaire is widely used in management, professional selection and career guidance, in law enforcement agencies, in the practice of clinical psychologists and in education. Method category: Personality questionnaire Application of the method Child version (CPQ) - from 7 to 12 years Adolescent version (HSPQ) - from 12 to 16 years Adult version (16PF) - from 16 years Testing time: 40–50 minutes Form of administration: Individual, group, computer individual Results processing: Manual, computer

Slide 24

Rosenzweig's frustration test

Purpose of the technique The test is designed to identify emotional response patterns in stressful situations and predict behavior in interpersonal interactions. Application of the technique Age range: Children's version - from 7 to 14 years Adult version - from 14 years Testing time: 25-30 minutes Form of implementation: Individual Processing of results: Manual, computer L. Ya. Yasyukova adapted the adult and children's version of the “Frustration Test” technique S. Rosenzweig."

Slide 25

Wechsler test (child version)

Purpose of the technique Category of the technique: Cognitive test The technique allows you to measure the level of development of general, verbal and non-verbal intelligence, private intellectual abilities; identify learning potential; determine the level of intellectual integrity. Application of the technique Age range: Children's version - from 5 to 16 years old Adult version - from 16 years of age Testing time: 90-100 minutes Form of implementation: Individual Processing of results: Manual

Slide 26

Diagnostics of differentiation of the child’s emotional sphere “Houses” (Methodology of O.A. Orekhova)

Purpose of the technique Category of the technique: Psychosemantic The technique can be used in psychological counseling and psychotherapy to predict difficulties in the development of the emotional sphere and develop correction programs for the personal characteristics of children. Application of the methodology Age range: From 4 to 12 years Testing time: 20 minutes Form of implementation: Individual, group, computer-based individual Processing of results: Manual, computer

Slide 27

Slide 28

Bibliography

Slide 29

M.V.Gamezo, E.A.Petrova, L.M.Orlova

AGE AND PEDAGOGICAL PSYCHOLOGY Mikhail Viktorovich Gamezo - professor, doctor of psychological sciences, author of about 100 scientific works, one of the founders of the psychosemiotic approach of modern Russian psychology. His most famous books are “Atlas of Psychology” and “Course of Psychology” (in 3 parts). Mikhail Viktorovich Gamezo was awarded the badges “Excellence in Education of the USSR”, “Excellence in Education of the RSFSR”, and the medal K.D. Ushinsky and a silver medal from VDNKh. For a long time he headed the Department of Psychology at Moscow State Pedagogical University. M.A. Sholokhov, where he continues to work as a consulting professor. Elena Alekseevna Petrova - professor, doctor of psychological sciences, author of more than 120 scientific and popular science works, the most famous of which are “Gestures in the pedagogical process”, “Signs of communication”, etc. Elena Alekseevna Petrova is an honorary worker of the Russian higher professional education system Federation, head of the Department of Social Psychology at MGSU, Professor at the Department of Psychology at MGOPU. Lyubov Mikhailovna Orlova - associate professor, candidate of psychological sciences, specialist in the field of history of psychology, psychology of communication, author of many scientific and educational works, the most famous of which are “Psychodiagnostics of preschoolers and younger schoolchildren”, “Age psychology: personality from youth to old age” " Veteran of labour.

Slide 30

ELKONIN Daniil Borisovich

Soviet psychologist, who was part of the backbone of the scientific school of L.S. Vygotsky The author owns remarkable theories of the periodization of child development and children's play, as well as methods of teaching children to read. Studied at the Leningrad Pedagogical Institute. A. I. Herzen. Since 1929 he worked at this institute; For several years, in collaboration with L. S. Vygotsky, he studied the problems of children's play. D. B. Elkonin is the author of several monographs and many scientific articles devoted to the problems of the theory and history of childhood, its periodization, the mental development of children of different ages, the psychology of play and learning activities, psychodiagnostics, as well as issues of child speech development and teaching children to read. List of the main scientific works of D. B. Elkonin: Thinking of a junior schoolchild / Essays on the psychology of children. M., 1951; Child psychology. M., 1960; Primer (experimental). M., 1961; Questions of psychology of educational activity of junior schoolchildren / Ed. D. B. Elkonina, V. V. Davydova. M., 1962; Intellectual capabilities of younger schoolchildren and the content of education. Age-related opportunities for acquiring knowledge. M., 1966; Psychology of teaching primary schoolchildren. M., 1974; How to teach children to read. M., 1976;

Slide 31

Vygotsky L.S.

Cultural-historical concept of mental development. He introduced a new experimental-genetic method for studying mental phenomena, since he believed that “the problem of the method is the beginning and basis, the alpha and omega of the entire history of the cultural development of the child.” L.S. Vygotsky developed the doctrine of age as a unit of analysis of child development. He proposed a different understanding of the course, conditions, source, form, specificity and driving forces of the child’s mental development; described the eras, stages and phases of child development, as well as transitions between them during ontogenesis; he identified and formulated the basic laws of the child’s mental development. According to L.S. Vygotsky, the driving force of mental development is learning. It is important to note that development and learning are different processes. The concept of the zone of proximal development has important theoretical significance and is associated with such fundamental problems of child and educational psychology as the emergence and development of higher mental functions, the relationship between learning and mental development, driving forces and mechanisms of mental development child. 1935 Mental development of children in the learning process. [Sat. articles] State-educational. teacher, ed., Moscow. 1982-1984 Collected works in 6 volumes. (Vol. 1: Questions of the theory and history of psychology; Vol. 2: Problems of general psychology; Vol. 3: Problems of mental development; Vol. 4: Child psychology; Vol. 5: Fundamentals of defectology; Vol. 6: Scientific heritage). Pedagogy, Moscow. 1956 Thinking and speech. Problems of child psychological development. Selected pedagogical studies, Publishing House of the Academy of Pedagogical Sciences of the RSFSR. Moscow.

Slide 32

Leontyev A.N.

Developed in the 20s. together with L.S. Vygotsky and A.R. Luria cultural-historical theory, conducted a series of experimental studies revealing the mechanism of formation of higher mental functions (voluntary attention, memory) as a process of “growing”, interiorization of external forms of instrumentally mediated actions into internal mental processes. Experimental and theoretical works are devoted to problems of mental development (its genesis, biological evolution and socio-historical development, development of the child’s psyche), problems of engineering psychology, as well as the psychology of perception and thinking. The concept of Leontiev’s activity was developed in various branches of psychology (general, child, developmental, pedagogical, medical, social), which in turn enriched it with new data. The position formulated by Leontyev on leading activity and its determining influence on the development of the child’s psyche served as the basis for the concept of periodization of children’s mental development, put forward by D.B. Elkonin. Works: Selected psychological works, vol. 1-2.- M., 1983; Sensation, perception and attention of children of primary school age // Essays on the psychology of children (junior school age). - M., 1950; Child's mental development. - M., 1950; Category of activity in modern psychology // Questions of psychology, 1979, No. 3.

Slide 33

Kudryavtsev V.T.

Doctor of Psychology, Professor, Head of the Laboratory of Psychological and Pedagogical Foundations of Developmental Education of the Russian Academy of Education. Raises questions about developmental education, about the continuity of the preschool and primary school levels. The problem of continuity of educational levels becomes particularly acute at the turning point of preschool and primary school age. The fact is that there is a radical change in the social situations of children's development - from communicative and playful to educational. In the context of this contradiction, the problem of continuity of preschool and primary education is considered in the works of L.S. Vygotsky, D.B. Elkonina. under the leadership of V.V. Davydov and V.T. Kudryavtsev, special design and research work was launched to create an appropriate succession model. This work has been carried out since 1992 on the basis of the Moscow School-Laboratory “Losiny Ostrov” No. 368, which includes preschool and school levels (the latter uses developmental education technologies in its activities according to the system of D.B. Elkonin - V.V. Davydov). Currently, similar experimental sites have been created in a number of regions of Russia. Record-Start program. The goal of the Project is to create conditions that ensure the general mental development of children aged 3-6 years by means of developing their imagination and other creative abilities, in particular, as a condition for the formation of their future ability to learn. The set goal is dictated by the following tasks of the project: initiation and psychological and pedagogical support of the processes of creative development of culture by children within the framework of various types of their activities (games, artistic and aesthetic activities, teaching, etc.); development of the creative imagination of preschoolers, the system of the child’s creative abilities based on it (productive thinking, reflection, etc.), creativity as the leading property of his personality; development and maintenance of specific cognitive motivation and intellectual emotions in children; expanding the prospects for child development by including preschoolers in developmental forms of joint activities with adults and with each other; cultivating in children a creative value attitude towards their own physical and spiritual health.

Slide 34

Literature

Vygotsky L.S. Collection op. in 6 volumes. T. 5. M.: Pedagogika, 1983. P. 153-165 Vygotsky L.S. 1982-1984 Collected works in 6 volumes. (Vol. 1: Questions of the theory and history of psychology; Vol. 2: Problems of general psychology; Vol. 3: Problems of mental development; Vol. 4: Child psychology; Vol. 5: Fundamentals of defectology; Vol. 6: Scientific heritage). Pedagogy, Moscow. Gamezo M.V., Petrova E.A., Orlova L.M. Age and educational psychology: Textbook. a manual for students of all specialties of pedagogical universities. - M.: Pedagogical Society of Russia, 2003. - 512 p. G. Craig, D. Brown “Developmental Psychology” 9th edition, publishing house “Peter” Questions of psychology of educational activity of younger schoolchildren / Ed. D. B. Elkonina, V. V. Davydova. M., 1962; Thinking of a primary school student / Essays on the psychology of children. M., 1951; Child psychology. M., 1960; Primer (experimental). M., 1961;

View all slides

Summary

Organization of education for children in primary school. The objective nature of the difficulties that a child faces at the beginning of school. The main problems of the adaptation period: inclusion in a new activity, entering a new system of relationships, getting used to an unusual daily routine and work, the emergence of new responsibilities, the need to demonstrate such personality qualities as discipline, responsibility, perseverance, perseverance, efficiency and hard work. Ways to overcome the difficulties of the adaptation period to school. Additional moral stimulation of the child for success. Formation of the main components of educational activities: educational actions, actions to control and evaluate work results. Causes of intellectual passivity and retardation of children in primary school, ways to eliminate them. Group forms of organizing classes in the first months of school.

Teaching primary schoolchildren at home. The special importance of home study work with first-graders. Formation of independent learning activities. Development of speech and thinking through improving writing. Presentation, retelling of what was read, seen or heard, writing letters and short essays are the main means of speech development. Two main directions for improving the theoretical and practical thinking of junior schoolchildren. The role of mathematical, linguistic exercises, and everyday tasks in improving a child’s thinking. Various types of creative activities: designing, drawing, modeling - as a means of improving practical and visual-figurative thinking.

Play and work activities in primary schoolchildren. Changing the nature of children's games at primary school age. The emergence and spread of competitive games and construction games that promote the development of business intellectual qualities in children. Accustoming a child to work. Developmental significance of children's sports games. Developmental types of work activity. Organization of child labor at school and at home. Labor as initiative, independent and creative work.

Sources of mental development of children of primary school age. Print, radio, television, various types of art as sources of intellectual development for children of primary school age. Fine art as a means of developing and enriching the perception of the world, as a way of getting rid of an egocentric point of view. Developing the child’s ability to correctly understand and accept someone else’s point of view. The art of cinema and television as a means of expanding and deepening the vision of the world. Developmental opportunities of the theater. The role of literature and periodicals in the intellectual development of children. The need for reading as a means of improving verbal thinking. Reasons for the learning lag in children of primary school age. Learning ability and level of mental development of the child. Age-related learning capabilities. Weakness of memory as one of the reasons for children to lag behind in learning. Symbolic encoding and cognitive organization of material to improve memory. Psychological and pedagogical analysis of the reasons for the lag in learning of children of primary school age.

ORGANIZATION OF TEACHING CHILDREN IN JUNIOR SCHOOL GRADES

Regardless of how much effort and time is spent on ensuring that children are ready for school in preschool age, almost all children face certain difficulties during the initial period of education. Therefore, there is a transition period from preschool to school childhood, which can be called the period of adaptation of the child to school. For a general psychological description of this and subsequent periods in a child’s life associated with radical changes in his psychology and behavior, it is useful to use the concepts social situation of development and internal position. The first of these concepts relates to the social conditions in which the process of mental development of the child takes place. It also includes an idea of ​​the place occupied by the child in society, in the system of division of labor, and the rights and responsibilities associated with this. The second concept characterizes the child’s inner world, the changes that must occur in it so that the child can adapt well to the new social situation and use it for his further psychological growth. These changes are usually associated with the formation of new relationships, new meaning and purpose in life, affecting needs, interests and values, forms of behavior and attitudes towards people. In general, they are also associated with the beginning of serious personal and interpersonal changes in the child’s psychology.

There are relatively few such moments in a person’s life when profound changes in the social situation of development occur. This is entering school, graduating from it, obtaining a profession and starting independent work, forming a family, transitions from one age to another: from 20-25 to 40-50 years, from 40-50 years to the age of 60 years, step by step. limits of 70 years of age.

What's the best way to do this? First of all, it is necessary to pay attention to the formation of full-fledged educational activities in first-graders. The main parameters, signs and methods for assessing the degree of development of this activity were described in the previous section of the textbook. Let's add something that directly concerns first-graders. Psychological and pedagogical analysis shows that they most often encounter two types of difficulties: fulfilling the Regimen and entering into new relationships with adults. The most common negative phenomenon at this time is satiety with classes, which quickly sets in for many children soon after they enter school. Outwardly, it usually manifests itself in the inability to maintain the initial natural interest in school and academic subjects at the proper level.

To prevent this from happening, it is necessary to include additional incentives for educational activities. When applied to children aged six or seven, such incentives can be both moral and material. Moral incentives It is no coincidence that they are put in first place here, since in stimulating children of primary school age to learn, they often turn out to be more effective than material ones. These include, for example, approval, praise, setting the child as an example to other children. It is important, by carefully observing the child’s behavior, to notice in time what he responds best to, and more often to turn to forms of moral encouragement associated with this in the early stages of schooling, it is advisable to exclude or minimize any punishments for poor studies. As for material incentives for success, then, as practice shows, they are pedagogically and psychologically ineffective and act mainly situationally. They can be used, but they cannot be abused. At the same time, it is necessary to combine material and moral ways of stimulating the child’s learning.

Initially, the teaching process in the lower grades of school is built on the basis of familiarizing children with the main components of educational activity. These components, according to V.V. Davydov, are the following: learning situations, learning actions, control and evaluation. It is necessary to demonstrate to children in detail and slowly a certain sequence of educational actions, highlighting among them those that must be performed in the subject, external speech and mental planes. At the same time, it is important to create favorable conditions so that objective actions acquire mental form with proper generalization, abbreviation and mastery.

In educational situations, children master general methods of solving a certain class of problems, and the reproduction of these methods acts as the main goal of educational work. Having mastered them, children immediately and fully apply the found solutions to specific problems that they encounter.

Actions aimed at mastering a general pattern - a method for solving a problem - are motivated accordingly. The child is explained why he needs to learn this particular material.

Work on mastering general patterns of action should precede the practice of using them in solving specific problems and stand out as special in the educational process. One of the main requirements of psychology is to organize initial education in such a way that the teaching of most topics and sections of the program takes place on the basis of educational situations that orient children toward mastering general ways of identifying the properties of a certain concept or general patterns of solving problems of a certain class. Research shows that a number of significant shortcomings in mastering certain concepts and methods of solving problems are associated with the fact that when developing these concepts and methods of solving problems, children were not trained to perform all the necessary educational actions.

The ability to transform concrete practical problems into educational and theoretical ones demonstrates the highest level of development of schoolchildren’s educational activities. If this skill is not properly developed at primary school age, then subsequently neither diligence nor conscientiousness can become a psychological source of successful learning. The need for control and self-control in educational activities creates favorable conditions for the development in younger schoolchildren of the ability to plan and perform actions silently, internally, as well as to regulate them voluntarily.

Spontaneous reasoning out loud helps children develop thinking and speech. In one experiment, a group of 9-10 year old children were taught to reason out loud while performing a task. The control group did not receive such experience. Children from the experimental group completed the intellectual task much faster and more efficiently than children from the control group. The need to reason out loud and justify one’s decisions leads to the development of reflexivity as an important quality of the mind, allowing a person to analyze and understand his judgments and actions. There is a development of voluntary attention, transformation of memory processes on an arbitrary and meaningful basis. At the same time, voluntary and involuntary types of memory interact and contribute to the development of each other.

The mental abilities and ability to master educational material by younger schoolchildren are quite high. With properly organized training, children perceive and learn more than what a regular school traditionally provides. The first thing you need to teach a younger student when doing homework is to identify a learning task. The child must clearly understand what method of performing a task he needs to master, why this or that task is needed as a learning task, and what it can teach.

Good results in teaching primary school children are achieved by group forms of organizing classes, reminiscent of role-playing games, to which children are accustomed in preschool age and in which they participate with pleasure. At the beginning of school, it is recommended to organize joint, group learning activities. However, this form of management, especially in the first months of children’s schooling, requires careful preparation. One of the main tasks that needs to be solved when starting group training is to correctly distribute roles and establish an atmosphere of friendly interpersonal relationships based on mutual assistance in the training group.

The problem of learning and mental development is one of the oldest psychological and pedagogical problems. There is, perhaps, not a single significant didactic theorist or child psychologist who would not try to answer the question of the relationship between these two processes. The issue is complicated by the fact that the categories of training and development are different. The effectiveness of teaching, as a rule, is measured by the quantity and quality of acquired knowledge, and the effectiveness of development is measured by the level that the students’ abilities reach, i.e., by how developed the students’ basic forms of mental activity are, allowing them to quickly, deeply and correctly navigate the phenomena of the environment reality.

It has long been noted that you can know a lot, but at the same time not show any creative abilities, that is, not be able to independently understand a new phenomenon, even from a relatively well-known field of science.

Progressive teachers of the past, especially K. D. Ushinsky,

raised and resolved this question in their own way. K. D. Ushinsky especially advocated that education be developmental. Developing a method of teaching primary literacy, new for his time, he wrote: “I do not prefer the sound method because children learn to read and write more quickly with it; but because, while successfully achieving its special goal, this method at the same time gives independent activity to the child, constantly exercises the child’s attention, memory and reason, and when a book is then opened in front of him, he is already significantly prepared to understand what he is reading, and, most importantly, his interest in learning is not suppressed, but rather aroused” (1949, vol. 6, p. 272).

During the time of K.D. Ushinsky, the penetration of scientific knowledge into primary school programs was extremely limited. That is why then there was a tendency to develop the child’s mind on the basis of mastering not scientific concepts, but special logical exercises, which were introduced into primary education by K. D. Ushinsky. By this, he sought to at least to some extent compensate for the lack of mental development based on existing programs that limited training to purely empirical concepts and practical skills.

To this day, such exercises are used when teaching language. By themselves, they have no developmental significance. Typically, logic exercises come down to classification exercises. Since in this case the household objects surrounding the child are subject to classification, it is, as a rule, based on purely external signs. For example, children divide objects into furniture and dishes or vegetables and fruits. When classifying an item as furniture, it is essential that these are furnishings, and as utensils, they are used for preparing food or consuming it. The concept of “vegetables” includes both fruits and roots; thereby removing the essential features of these concepts, based on external properties or methods of use. Such a classification can have an inhibitory effect during the subsequent transition to scientific concepts proper, fixing the child’s attention on the external signs of objects.

As primary education programs become saturated with modern scientific knowledge, the importance of such formal logical exercises decreases. Although to this day there are still teachers and psychologists who believe that exercises in mental operations on their own are possible, regardless of the content material.

The development of a developmental training system is based on the solution of a more general problem of training and development. Although the very formulation of the question of developmental training already presupposes that training has a developmental significance, the specific content of the relationship between training and development requires its disclosure.

Currently, there are two main ones in a certain

sense, opposite points of view on the relationship between training and development. According to one of them, presented mainly in the works of J. Piaget, development and mental development do not depend on learning. Education is considered as an external intervention in the development process, which can influence only some features of this process, somewhat delaying or accelerating the appearance and time course of individual regularly changing stages of intellectual development, but without changing either their sequence or their psychological content. With this point of view, mental development occurs within the child’s system of relationships with the things around him as physical objects.

Even if we assume that there is such a direct collision of a child with things, which occurs without any participation of adults, then in this case there is a peculiar process of acquiring individual experience, which has the character of spontaneous, unorganized self-learning. In reality, such an assumption is an abstraction. The fact is that the things surrounding the child do not have their social purpose written on them, and the method of their use cannot be discovered by the child without the participation of adults. The bearers of social ways of using and consuming things are adults, and only they can pass them on to a child.

It is difficult to imagine that a child, on his own, without any interference from adults, would go through the path of all the inventions of mankind in the period of time provided to him by childhood. A period that, compared to the history of mankind, is determined by an instant. There is nothing more false than the understanding of a child as a little Robinson, left to his own devices in the uninhabited world of things. The moral of the wonderful novel about Robinson Crusoe is precisely that a person’s intellectual power consists of those acquisitions that he brought with him to the desert island and which he received before he found himself in an exceptional situation; The pathos of the novel is in demonstrating the social essence of man even in an atmosphere of almost complete loneliness.

According to the second point of view, mental development occurs within the relationship between the child and society, in the process of assimilation of the generalized experience of humanity, fixed in a variety of forms: in the objects themselves and the ways of their use, in the system of scientific concepts with methods of action fixed in them, in the moral rules of relationships between people, etc. Education is a specially organized way of transmitting the social experience of humanity to an individual. While individual in its form, it is always social in content. Only this point of view can serve as the basis for developing a system of developmental education.

Recognition of the leading role of training for mental development in general, for mental development in particular, does not at all mean recognition that all training determines development. The very formulation of the question about developmental training, about the relationship between training and development, suggests that training can be different. Learning can determine development and can be completely neutral in relation to it.

Thus, learning to type on a typewriter, no matter how modern it is done, does not introduce anything fundamentally new into mental development. Of course, a person acquires a number of new skills, he develops flexibility of fingers and speed of orientation in the keyboard, but the acquisition of this skill does not have any effect on mental development.

What aspect of learning is decisive for mental development in primary school age? To answer this question, first of all, it is necessary to find out what is most important in the mental development of a junior schoolchild, that is, which aspect of his mental development needs to be improved so that it all rises to a new, higher level.

Mental development includes a number of mental processes. This is the development of observation and perception, memory, thinking and, finally, imagination. As follows from special psychological studies, each of these processes is connected with the others. However, the connection is not constant throughout childhood: in each period, one of the processes is of leading importance for the development of the others. Thus, in early childhood, the development of perception becomes of primary importance, and in preschool age, memory. It is well known with what ease preschoolers memorize various poems and fairy tales.

By the beginning of primary school age, both perception and memory have already gone through quite a long path of development. Now, for their further improvement, it is necessary that thinking rise to a new, higher level. By this time, thinking had already passed the path from practically effective, in which solving a problem is possible only in a situation of direct actions with objects, to visual-figurative, when the task does not require real action with objects, but tracing a possible solution path in a directly given visual field or in terms of visual representations preserved in memory.

The further development of thinking consists in the transition from visual-figurative to verbal-logical reasoning thinking. The next step in the development of thinking, which occurs already in adolescence and consists in the emergence of hypothetical-reasoning thinking (i.e. thinking that is built on the basis of hypothetical assumptions and circumstances), can

occur only on the basis of relatively developed verbal and logical thinking.

The transition to verbal-logical thinking is impossible without a radical change in the content of thinking. Instead of concrete ideas that have a visual basis, concepts must be formed whose content is no longer the external, concrete, visual signs of objects and their relationships, but the internal, most essential properties of objects and phenomena and the relationships between them. It must be borne in mind that the forms of thinking are always in organic connection with the content.

Numerous experimental studies indicate that along with the formation of new, higher forms of thinking, significant shifts occur in the development of all other mental processes, especially in perception and memory. New forms of thinking become means of carrying out these processes, and the re-equipment of memory and perception raises their productivity to greater heights.

Thus, memory, which in preschool age was based on emotional empathy for the hero of a fairy tale or on visual images that evoke a positive attitude, turns into semantic memory, which is based on the establishment of connections within the memorized material, semantic and logical connections. Perception from the analyzing, based on obvious signs , turns into establishing connections, synthesizing. The main thing that happens with the mental processes of memory and perception is their arming with new means and methods, which are formed primarily within problems solved by verbal-logical thinking. This leads to the fact that both memory and perception becomes much more manageable, for the first time it becomes possible to choose means for solving specific problems of memory and thinking.Means can now be selected depending on the specific content of the problems.

For memorizing poems, it is essential to comprehend each word used by the poet, and for memorizing the multiplication table, establishing functional relationships between the work and the factors when one of them is increased by one.

Thanks to the transition of thinking to a new, higher level, a restructuring of all other mental processes occurs, memory becomes thinking, and perception becomes thinking. The transition of thinking processes to a new stage and the associated restructuring of all other processes constitute the main content of mental development in primary school age.

Now we can return to the question of why training may not be developmental. This can happen when it is focused on already developed forms of mental activity of the child - perception, memory and forms of visual

figurative thinking characteristic of the previous period of development. Training structured in this way reinforces the already completed stages of mental development. It trails behind development and therefore does not move it forward.

An analysis of the content of our elementary school programs shows that they have not completely eliminated the goal of children acquiring empirical concepts and basic knowledge about the environment, practical skills in reading, counting and writing, which were characteristic of elementary school when it was a relatively closed cycle, and was not the initial link in the system of universal complete secondary education.

Let us return to the question of which aspect of learning is decisive for mental development in primary school age. Where lies the key, using which you can significantly strengthen the developmental function of education, solve the problem of the correct relationship between learning and development in the lower grades of school?

Such a key is the assimilation of a system of scientific concepts already at primary school age. The development of abstract verbal-logical thinking is impossible without a radical change in the content with which thought operates. The content in which new forms of thought are necessarily present and which necessarily requires them are scientific concepts and their system.

From the totality of social experience accumulated by mankind, school education should convey to children not just empirical knowledge about the properties and methods of acting with objects, but the experience of mankind’s knowledge of the phenomena of reality, generalized in science and recorded in the system of scientific concepts: nature, society, thinking.

It must be especially emphasized that the generalized experience of cognition includes not only ready-made concepts and their system, a method of their logical ordering, but - and this is especially important - the methods of action behind each concept through which this concept can be formed. In a certain way, didactically processed generalized methods of analyzing reality characteristic of modern science, leading to the formation of concepts, should be included in the content of training, constituting its core.

The content of learning should be seen as a system of concepts about a given area of ​​reality to be mastered, together with the methods of action through which concepts and their system are formed in students. Concept - knowledge about the essential relationships between individual aspects of an object or phenomenon. Consequently, in order to form a concept, it is necessary first of all to highlight these aspects, and since they are not given in direct perception, it is necessary to carry out completely definite, unambiguous, concrete actions with objects in order to

properties appeared. Only by highlighting the properties can one determine in what relationships they are located, but to do this they must be placed in different relationships, i.e., be able to change relationships. Thus, the process of concept formation is inseparable from the formation of actions with objects that reveal their essential properties.

Let us emphasize once again: the most important feature of mastering concepts is that they cannot be memorized, you cannot simply tie knowledge to the subject. The concept must be formed, and it must be formed by the student under the guidance of the teacher.

When we gave the child the word “triangle” and told him that it is a figure consisting of three sides, we told him only the word for naming the object and its most general characteristics. The formation of the concept of “triangle” begins only when the child learns to relate its individual properties - its sides and angles (when the student establishes that in this figure the sum of two sides is always greater than the third, that the sum of the angles in it is always equal to two right angles, that The larger angle always lies opposite the larger side, etc.). A concept is a set of definitions, a set of many essential relations in an object. But not one of these relationships is given in direct observation; each of them must be discovered, and it can only be discovered through actions with the object.

Actions with objects, through which their essential properties are revealed and essential relationships between them are established, are the ways in which our thinking works. Already in initial education, it is especially important to establish the relationships between individual aspects of objects or phenomena of reality. There are endless possibilities for this - both in teaching mathematics and in teaching language.

If we teach children the number series, then it is necessary to achieve understanding and establish the relationships between the numbers included in it, and perhaps derive a general formula for its construction. If we introduce a child to the decimal number system, then it is necessary to identify the essential relationship on the basis of which it is built and show that it is not the only possible one. When we introduce children to arithmetic operations, it is especially important to establish significant relationships between the elements included in their structure. If we teach a child to read and write, then the most important thing is to establish the relationship between the phonemic structure of the language and its graphic designations. When we introduce children to the morphological structure of a word, we need to find out the system of relationships between the main and additional meanings in the word. The number of such examples could be multiplied ad infinitum.

It is essential, however, not just the formation of individual concepts, but the creation of their system. True, science itself helps with this, which is necessarily a system of concepts, where each concept is connected with others. Logical reasoning, - with one

on the one hand, reasoning about the relationship between individual aspects in a subject, and on the other hand, reasoning about the connections between concepts. Movement in the logic of these connections is the logic of thinking. Thus, we have found the key to the problem of developmental education in primary school age. This key is the content of the training. If we want education in the primary grades of school to become developmental, then we must take care, first of all, that the content is scientific, that is, that children learn the system of scientific concepts and how to obtain them. The development of children's thinking during this period is the key to their overall mental development.

Sections: Primary School

Our children are smarter the more opportunities we give them to be smart.

Glen Doman.

Nowadays, the problem of developmental education for schoolchildren is again relevant. . Again, because the idea of ​​child development was fundamental to the Russian public school of the second half of the 19th - early 20th centuries.

“A child who has completed a primary school course must be able to work not only with his memory, he must acquire some development that will give him the opportunity... to use a book and through it to acquire knowledge.... Primary school will not give you development, but will only give you a supply of information - this information will certainly be uselessly memorized: if the school does not make you think...”

Attention to the problem of a child’s intellectual development is dictated by the conditions of modern life.

A person’s entire life constantly confronts him with acute and urgent tasks and problems. The emergence of such problems, difficulties, and surprises means that in the reality around us there is still a lot of unknown, hidden things. Consequently, we need an ever deeper knowledge of the world, the discovery in it of more and more new processes, properties and relationships of people and things. Therefore, no matter what new trends, born of the demands of the time, penetrate the school, no matter how the programs and textbooks change, the formation of a culture of intellectual activity of students has always been and remains one of the main general educational and educational tasks. Intellectual development is the most important aspect of preparing younger generations.

The success of a student's intellectual development is achieved mainly in the classroom, when the teacher is left alone with his students. And the degree of students’ interest in learning, level of knowledge, readiness for constant self-education, i.e. depends on his ability to “fill the vessel and light the torch,” and his ability to organize systematic cognitive activity. their intellectual development, which is convincingly proven by modern psychology and pedagogy.

Most scientists recognize that the development of schoolchildren’s creative abilities and intellectual skills is impossible without problem-based learning.

Creative abilities are realized through mental activity.

The psychological basis of the concept of problem-based learning is the theory of thinking as a productive process, put forward by S.L. Rubinstein. Thinking plays a leading role in human intellectual development.

Significant contributions to the disclosure of the problem of intellectual development, problem-based and developmental learning were made by N.A. Menchinskaya, P.Ya. Galperin, N.F. Talyzina, T.V. Kudryavtsev, Yu.K. Babansky, I.Ya. Lerner, M I. Makhmutov, A. M. Matyushkin, I. S. Yakimanskaya and others.

Although this problem is discussed in sufficient detail in the psychological, pedagogical and methodological literature, it has not received due attention in school practice.

The developmental education system is aimed at developing children's intellectual abilities, desires and ability to learn, and skills of business cooperation with peers. At primary school age, the child experiences intensive development of intelligence. At the same time, it is necessary to remember that intellectual abilities develop through activity and that their development requires high cognitive activity of children. Moreover, not every activity develops abilities, but only emotionally pleasant ones.

Even Jan Amos Komensky called for making the work of a schoolchild a source of mental satisfaction and spiritual joy. Since then, every progressively thinking teacher considers it necessary for the child to feel: learning is a joy, and not just a duty; learning can be done with passion. Therefore, lessons must be at a high level of interest and cognitive activity, take place in a friendly environment and in a situation of success.

The effectiveness of the intellectual development of primary schoolchildren depends on the activities of the teacher, his creative approach to teaching children, when the teacher gives preference to teaching methods and techniques that stimulate complex cognitive processes and promotes independent activity of students, focused on their creativity. The formation of a harmonious mindset is one of the main tasks of the pedagogical process.

The educational material must be problematic in nature. The tasks offered to students must present a problem-solving task. Such a task is an artificial pedagogical construction, since the educational process uses those problematic tasks that have already been solved by society and the teacher already knows this solution. For the student, the task acts as a subjective problem.

If the educational material is problematic in nature, and children do not have the basis for solving an abstract-mental creative problem, then in this case the teacher must construct the task in such a way that the conditions of the task become accessible to the direct perception of the students or can be visually represented by them.

Spelling of unstressed vowels at the root of a word

2nd grade (1 - 4)

Goals:

• To consolidate students' knowledge of spelling words with an unstressed vowel at the root.
• To develop the ability to justify the choice of unstressed vowels when writing words.
• Develop speech, thinking, attention, memory of students.
• Cultivate interest in the Russian language.

I. Warm-up.

The exercises are performed to the soundtrack of birdsong. Doing exercises for brain activity and preventing visual impairment is an important part of the training. Research by scientists proves that under the influence of physical exercise, the performance of various mental processes underlying creative activity improves: memory capacity increases, attention stability increases, the solution of elementary intellectual problems is accelerated, and psychomotor processes are accelerated. (cm. Application )

II. Formulating the topic of the lesson.

Spring is a special time of year. Awakened by warmth and light, nature awakens. Life seems to be born again. We are looking forward to spring! In Rus' they called for spring and sang songs to it. Spring is the morning of the year!

– What did you read about? How do you understand the last lines?
– Which word did you come across more often than others in the text? (Spring)
– Why is one sound heard in the word spring, but a different vowel is written? (The letter is in a weak position; its spelling needs to be checked.)
– Determine in which part of the word the letter is missing? Prove it. (Freckle, spring)
– Formulate the topic of our lesson.
– How to check the unstressed vowel at the root of a word?

On the board: SPRING - SPRING.

III. Repetition of learned material.

On the board: R..DOK, V..DRO, BIRCH, ROV..R, GR..ZA, SPOS..B, ST...NAL, RASPBERRY, GREEN..NY.

– Read the words, divide them into two groups according to two characteristics at the same time.
– What groups did you get?
– Why are the letters in the words of the first column missing, but not in the second column?
– In which part of the word are the letters missing?
– What should you do to correctly write an unstressed vowel at the root of a word?
– Look at the algorithm for selecting related words.

• One is many
• Many - one
• Call me kindly
• Find the root
• Choose another part of speech.

IV. Working with signal cards.

What unstressed vowel should we insert into the root of the word ROW? BUCKET? COOK? STORM? WAY? MOANING? GREENERY? Prove using an algorithm for selecting related words.

V. A minute of penmanship.

– In a minute of penmanship, we will write letters that are untestable unstressed vowels in these words. What letters are these? ( e , A )
– Determine the order of letters in each chain: aae, abe, ave, age, ...
– Write this chain of letters in the order indicated until the end of the line.
– Write down words with an untestable unstressed vowel in your notebook (birch, raspberry)

VI. Vocabulary and spelling work.

– You will name the word we will learn about in class. To do this, connect the last letters of the words with the unstressed vowel being tested in the root, which you worked with while repeating what you learned. What word is this? (SHIP).
– Choose a generic concept for the word SHIP. (A ship is a transport)
-What is it intended for? (For transporting people and goods by water)
- Tell me in full, what is a ship? (A ship is a vehicle designed to transport people and goods by water.)
-What other ships are there? (Space. People fly into space on them)
– Look at the word SHIP. What can you say about its writing? (Unprov. unstressed vowel O, at the end b).
- Speak spellingly.
– Write this word in your notebook. Underline the unchecked unstressed vowel.
– Read the proverb written on the board. Explain its meaning.

For a big ship, a long voyage.

(A person with great abilities, with great talent must be given more opportunities so that he can develop them further and be able to achieve great success).

- Write the proverb from memory.
– What task can you offer with this proverb in accordance with the topic of the lesson? (Find words in the proverb with a verifiable b/gl in the root and check their spelling)

VII. Physical education minute.

Words: water, river, Steps, grass, forests, village, affairs, night, sweeps, terrestrial, seas, family.

VIII. Consolidation of what has been learned.

Exercise 1.

On the desk: house, domino, brownie, Houses, home, blast furnace, housewife.

- Read the words. What words are missing here? Why?
- Write down these words. What can you say about them?
– What words are called cognates?
– What can you say about the words house and home? (This is a form of the same word)
– Highlight the root in the words. Let's look at the vowel at the root. Does it sound the same in all words?
– When does a vowel sound clear and distinct? (Stressed)
– And when the sound is not heard clearly? (No accent, in weak position)
– Why in words brownie, Houses, home, housewife at the root it is written without accent O ? (The roots of words with the same root are written the same)
– The letter denoting an unstressed sound at the root of a word is an orthogram. We underline it with one line.

Exercise 2.

Work in pairs.

– There are two cards in front of you. On one are words with the unstressed vowel being tested, and on the other are words testing them. One student reads the word, the other looks for the test word. Together you write down a couple of words and highlight the spelling.

Checking what you have written.

– What did you remember when you did the exercise? Did you have any difficulties?

Exercise 3.

Textbook by A.V. Polyakova 2nd grade, p. 179, ex. 420.

– Find a sentence in the text, each word of which would have a tested unstressed vowel at the root of the word.
– How do you check an unstressed vowel at the root of a word?
– What words are test words?

IX. Lesson summary.

– What spelling did we work on in class today?
– What do you need to remember in order to correctly write an unstressed vowel at the root of a word?
– Can all related words be test words?

If the letter is a vowel
Raised doubts
You immediately
Put emphasis on it.

– Which proverb, in your opinion, would be suitable for today’s lesson?

(Signal pads)

• A mind is good, but two are better.
• Learning is always useful.
• Without flour there is no science.
• Where there is desire and patience, there is skill.

A soundtrack of birds singing sounds.

Literature

1. Russian primary teacher. – St. Petersburg, 1901 No. 1. – p. 5
2. Palamarchuk V.F. School teaches you to think. – M.: Education, 1987.
3. Doman G., Doman J. How to develop a child's intelligence. – M., 2000.
4. Bakulina G. A. Intellectual development of junior schoolchildren in Russian language lessons. – M., 2001.
5. Kholodova O. To young smart people and smart girls. Tasks for the development of creative abilities. – M., Rostkniga, 2002.
6. Development of students in the learning process: Ed. L.V. Zankova. – M., 1963.
7. Bogoyavlensky D.N., Menchinskaya N.A. Psychology of knowledge acquisition at school. – M., 1959.
8. Velichkovsky B.M. How natural intelligence works.//Nature. – 1988. – No. 12.

Leites N. S. Mental abilities and age. M. Pedagogy, 1971

Application

DEVELOPMENT OF JUNIOR SCHOOL CHILDREN IN THE PROCESS OF TEACHING MATHEMATICS

What is developmental education?

The term “developmental education” is actively used in psychological, pedagogical and methodological literature. However, the content of this concept still remains very problematic, and the answers to the question: “What kind of training can be called developmental?” quite contradictory. This, on the one hand, is due to the multifaceted nature of the concept of “developmental education”, and on the other hand, due to some inconsistency of the term itself, because One can hardly speak of “non-developmental education.” Undoubtedly, any training develops a child.

However, one cannot but agree that in one case, training is, as it were, built on top of development, as L.S. said. Vygotsky “lags behind” development, exerting a spontaneous influence on it; in another, he purposefully ensures it (leads development) and actively uses it to acquire knowledge, skills, and abilities. In the first case, we have the priority of the informational function of learning, in the second - the priority of the developmental function, which radically changes the structure of the learning process.

As D.B. writes Elkonin – the answer to the question of the relationship between these two processes “is complicated by the fact that the categories of training and development themselves are different.

The effectiveness of teaching, as a rule, is measured by the quantity and quality of acquired knowledge, and the effectiveness of development is measured by the level that the students’ abilities reach, i.e., by how developed the students’ basic forms of mental activity are, allowing them to quickly, deeply and correctly navigate the phenomena of the environment reality.

It has long been noted that you can know a lot, but at the same time not show any creative abilities, that is, not be able to independently understand a new phenomenon, even from a relatively well-known field of science.” .

It is no coincidence that methodologists use the term “developmental education” with great caution. Complex dynamic connections between the processes of learning and the mental development of a child are not the subject of research in methodological science, in which real, practical learning results are usually described in the language of knowledge, skills and abilities.

Since psychology studies the mental development of a child, when constructing developmental education, the methodology must undoubtedly be based on the results of research in this science. As V.V. Davydov writes, “the mental development of a person is, first of all, the formation of his activity, consciousness and, of course, all the mental processes that “serve” them (cognitive processes, emotions, etc.)” . It follows that the development of students largely depends on the activities that they perform during the learning process.

From the didactics course you know that this activity can be reproductive and productive. They are closely related, but depending on which type of activity predominates, learning has different effects on children's development.

Reproductive activity is characterized by the fact that the student receives ready-made information, perceives it, understands it, remembers it, and then reproduces it. The main goal of such activities is the formation of knowledge, skills and abilities in the student, the development of attention and memory.

Productive activity is associated with the active work of thinking and is expressed in such mental operations as analysis and synthesis, comparison, classification, analogy, generalization. These mental operations in psychological and pedagogical literature are usually called logical methods of thinking or methods of mental action.

The inclusion of these operations in the process of mastering mathematical content is one of the important conditions for building developmental education, since productive (creative) activity has a positive impact on the development of all mental functions. “... the organization of developmental education involves creating conditions for schoolchildren to master the techniques of mental activity. Mastering them not only provides a new level of assimilation, but also produces significant changes in the child’s mental development. Having mastered these techniques, students become more independent in solving educational problems and can rationally organize their activities to acquire knowledge.” .

Let's consider the possibilities of actively including various methods of mental action in the process of teaching mathematics.

3.2. Analysis and synthesis

The most important mental operations are analysis and synthesis.

Analysis is associated with the selection of elements of a given object, its characteristics or properties. Synthesis is the combination of various elements, aspects of an object into a single whole.

In human mental activity, analysis and synthesis complement each other, since analysis is carried out through synthesis, synthesis - through analysis.

The ability for analytical-synthetic activity is expressed not only in the ability to isolate the elements of an object, its various features or to combine elements into a single whole, but also in the ability to include them in new connections, to see their new functions.

The formation of these skills can be facilitated by: a) consideration of a given object from the point of view of various concepts; b) setting various tasks for a given mathematical object.

To consider this object from the point of view of various concepts, when teaching mathematics, primary schoolchildren are usually offered the following tasks:

Read the expressions 16 – 5 differently (16 is reduced by 5; the difference between the numbers 16 and 5; subtract 5 from 16).

Read the equality 15–5=10 differently (reduce 15 by 5, we get 10; 15 is greater than 10 by 5; the difference between the numbers 15 and 5 is 10;

15 – minuend, 5 – subtrahend, 10 – difference; if we add the subtrahend (5) to the difference (10), we get the minuend (15); the number 5 is less than 15 by 10).

What are different names for a square? (Rectangle, quadrilateral, polygon.)

Tell us everything you know about the number 325. (This is a three-digit number; it is written in numbers 3, 2, 5; it has 325 units, 32 tens, 3 hundreds; it can be written as a sum of digit terms like this: 300+20+5 ; it is 1 unit more than the number 324 and 1 unit less than the number 326; it can be represented as the sum of two terms, three, four, etc.)

Of course, you should not strive to ensure that every student pronounces this monologue, but, focusing on it, you can offer children questions and tasks, during which they will consider this object from different points of view.

Most often, these are tasks for classification or identifying various patterns (rules).

For example:

By what criteria can you separate buttons into two boxes?

Considering buttons from the point of view of their sizes, we will put 4 buttons in one box and 3 in another,

– in terms of color: 1 and 6,

– in terms of shape: 4 and 3.

Unravel the rule by which the table is compiled and fill in the missing cells:

Seeing that there are two rows in this table, students try to identify a certain rule in each of them, find out how much one number is less (more) than the other. To do this, they perform addition and subtraction. Having not found a pattern in either the top or bottom row, they try to analyze this table from a different point of view, comparing each number in the top row with the corresponding (below) number in the bottom row. Get: 4 8 to 1; 3>2 by 1. If under the number 8 we write the number 9, and under the number 6 – the number 7, then we have:

8 P for 1, P>4 for 1.

Similarly, you can compare each number in the bottom line with the corresponding (standing above it) number in the top line.

Such tasks with geometric material are possible.

Find the segment BC. What can you tell us about him? (BC – side of the triangle ALL; BC – side of the triangleDBC; Sun less thanDC; BC is less than AB; BC – side of the angleBCDand angle ALL).

How many segments are there in this drawing? How many triangles? How many polygons?

Consideration of mathematical objects from the point of view of various concepts is a way to compose variable tasks. Let’s take, for example, the following task: “Let’s write down all the even numbers from 2 to 20 and all the odd numbers from 1 to 19.” The result of its execution is the recording of two series of numbers:

2, 4, 6, 8, 10,12,14,16,18,20 1,3,5,7,9, 11, 13, 15, 17, 19

Now we use these mathematical objects to compose tasks:

Divide the numbers in each series into two groups so that each contains numbers that are similar to each other.

What is the rule for writing the first row? Continue it.

What numbers need to be crossed out in the first row so that each next one is 4 more than the previous one?

Is it possible to do this task for the second row?

Choose pairs of numbers from the first row whose difference is 10

(2 and 12, 4 and 14, 6 and 16, 8 and 18, 10 and 20).

Select pairs of numbers from the second row whose difference is 10 (1 and 11, 3 and 13, 5 and 15, 7 and 17, 9 and 19).

Which pair is “extra”? (10 and 20, there are two two-digit numbers in it, in all other pairs there is a two-digit number and a single-digit number).

Find in the first row the sum of the first and last numbers, the sum of the second numbers from the beginning and from the end of the series, the sum of the third numbers from the beginning and from the end of the series. How are these amounts similar?

Do the same task for the second row. How are the amounts received similar?

Task 80. Come up with tasks during which students will examine the objects given in them from different points of view.

3.3. Method of comparison

The technique of comparison plays a special role in organizing the productive activities of younger schoolchildren in the process of learning mathematics. The formation of the ability to use this technique should be carried out step by step, in close connection with the study of specific content. It is advisable, for example, to focus on the following stages:

highlighting features or properties of one object;

establishing similarities and differences between the characteristics of two objects;

identifying similarities between the characteristics of three, four or more objects.

Since it is better to begin the work of developing a logical method of comparison in children from the first lessons of mathematics, then as objects you can first use objects or drawings depicting objects that are familiar to them, in which they can identify certain features, based on the ones they have representation.

To organize student activities aimed at identifying the characteristics of a particular object, you can first ask the following question:

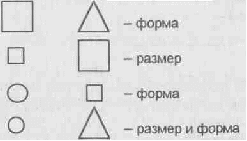
– What can you tell us about the subject? (The apple is round, large, red; the pumpkin is yellow, large, with stripes, with a tail; the circle is large, green; the square is small, yellow).

During the work, the teacher introduces children to the concepts of “size”, “shape” and asks them the following questions:

– What can you say about the sizes (shapes) of these objects? (Big, small, round, like a triangle, like a square, etc.)

To identify the signs or properties of an object, the teacher usually turns to children with questions:

– What are the similarities and differences between these items? - What changed?

It is possible to introduce them to the term “feature” and use it when performing tasks: “Name the characteristics of an object,” “Name similar and different characteristics of objects.”

Task 81. Select different pairs of objects and images that you can offer to first-graders so that they can establish the similarities and differences between them. Come up with illustrations for the task “What has changed...”.

Students transfer the ability to identify features and, based on them, to compare objects to mathematical objects.

V Name the signs:

a) expressions 3+2 (numbers 3, 2 and the “+” sign);

b) expressions 6–1 (numbers 6, 1 and the sign “–”);

c) the equality x+5=9 (x is an unknown number, numbers 5, 9, signs “+” and “=”).

Based on these external signs, accessible to perception, children can establish similarities and differences between mathematical objects and comprehend these signs from the point of view of various concepts.

For example:

What are the similarities and differences:

a) expressions: 6+2 and 6–2; 9 4 and 9 5; 6+(7+3) and (6+7)+3;

b) numbers: 32 and 45; 32 and 42; 32 and 23; 1 and 11; 2 and 12; 111 and 11; 112 and 12, etc.;

c) equalities: 4+5=9 and 5+4=9; 3 8=24 and 8 3=24; 4 (5+3)=32 and 4 5+4 3 = = 32; 3 (7 10) = 210 and (3 7) 10 = 210;

d) task texts:

Kolya caught 2 fish, Petya - 6. How many more fish did Petya catch than Kolya?

Kolya caught 2 fish, Petya - b. How many times more fish did Petya catch than Kolya? e) geometric figures:

f) equations: 3 + x = 5 and x+3 = 5; 10–x=6 and (7+3)–x=6;

12 – x = 4 and (10 + 2) – x = 3 + 1;

g) computational techniques:

9+6=(9+1)+5 and 6+3=(6+2)+1

L L

1+5 2+1

The comparison technique can be used when introducing students to new concepts. For example:

How are they all similar to each other?

a) numbers: 50, 70, 20, 10, 90 (tens place);

b) geometric figures (quadrangles);

c) mathematical notations: 3+2, 13+7, 12+25 (expressions called sums).

Task 82. Make up mathematical expressions from the given data:

9+4, 520–1.9 4, 4+9, 371, 520 1, 33, 13 1,520:1,333, 173, 9+1, 520+1, 222, 13:1 different pairs in which children can identify signs of similarities and differences. When studying which questions of a primary school mathematics course can each of your assignments be suggested?

In teaching primary schoolchildren, a large role is given to exercises that involve the translation of “subject actions” into the language of mathematics. In these exercises they usually correlate Object and symbolic objects. For example:

a) Which picture corresponds to the entries 2*3, 2+3?

b) Which picture corresponds to the entry 3 5? If there is no such picture, then draw it.

c) Complete the drawings corresponding to these entries: 3*7, 4 2+4*3, 3+7.

Task 83. Come up with various exercises for correlating subject and symbolic objects that can be offered to students when studying the meaning of addition, division, multiplication tables, division with a remainder.

The indicator of the formed™ method of comparison is the ability of children to independently use it to solve various problems, without instructions: “compare..., indicate the signs..., what are the similarities and differences...”.

Here are specific examples of such tasks:

a) Remove the sticky object... (When doing this, schoolchildren are guided by the similarities and differences of signs.)

b) Arrange the numbers in ascending order: 12, 9, 7, 15, 24, 2. (To complete this task, students must identify signs of differences between these numbers.)

c) The sum of the numbers in the first column is 74. How to find the sum of the numbers without performing addition in the second and third columns:

21 22 23

30 31 32

11 12 13

12 13 14 74

d)) Continue the series of numbers: 2, 4, 6, 8, ...; 1, 5, 9, 13, ... (The basis for establishing a pattern (rule) for writing numbers is also a comparison operation.)

Task 84. Show the possibility of using the comparison technique when studying addition of single-digit numbers within 20, addition and subtraction within 100, rules for the order of actions, as well as when introducing primary schoolchildren to rectangles and squares.

3.4. Classification method

The ability to identify the characteristics of objects and establish similarities and differences between them is the basis of classification.

From a mathematics course we know that when dividing a set into classes, the following conditions must be met: 1) none of the subsets is empty; 2) the subsets do not intersect pairwise;

3) the union of all subsets constitutes this set. When offering classification tasks to children, these conditions must be taken into account. Just as when developing the method of comparison, children first perform tasks to classify well-known objects and geometric figures. For example:

Students examine objects: cucumber, tomato, cabbage, hammer, onion, beetroot, radish. Focusing on the concept of “vegetable,” they can divide many objects into two classes: vegetables - non-vegetables.

Task 85. Come up with exercises of various contents with the instructions “Remove the extra object” or “Name the extra object”, which you could offer to students in 1st, 2nd, 3rd grade.

The ability to perform classification is developed in schoolchildren in close connection with the study of specific content. For example, for counting exercises, they are often given illustrations to which they can pose questions beginning with the word “How much...?” Let's look at the picture and ask the following questions:

- How many big circles? Little ones? Blue? Red? Big red ones? Little blue ones?

By practicing counting, students master the logical technique of classification.

Tasks related to the method of classification are usually formulated in the following form: “Divide (split) all the circles into two groups according to some criterion.”

Most children successfully complete this task, focusing on features such as color and size. As you learn different concepts, classification tasks may include numbers, expressions, equalities, equations, and geometric shapes. For example, when studying the numbering of numbers within 100, you can offer the following task:

Divide these numbers into two groups so that each contains similar numbers:

a) 33, 84, 75, 22, 13, 11, 44, 53 (one group includes numbers written with two identical digits, the other with different ones);

b) 91, 81, 82, 95, 87, 94, 85 (the basis of the classification is the number of tens, in one group of numbers it is 8, in another – 9);

c) 45, 36, 25, 52, 54, 61, 16, 63, 43, 27, 72, 34 (the basis of the classification is the sum of the “digits” with which these numbers are written, in one group it is 9, in another – 7 ).

If the task does not indicate the number of partition groups, then various options are possible. For example: 37, 61, 57, 34, 81, 64, 27 (these numbers can be divided into three groups, if you focus on the numbers written in the units place, and into two groups, if you focus on the numbers written in the tens place. Possible and another group).

Task 86. Make classification exercises that you could offer children to learn the numbering of five-digit and six-digit numbers.

When studying addition and subtraction of numbers within 10, the following classification tasks are possible:

Divide these expressions into groups according to some criteria:

a) 3+1, 4–1, 5+1, 6–1, 7+1, 8 – 1. (In this case, children can easily find the basis for dividing into two groups, since the attribute is presented explicitly in the expression record.)

But you can choose other expressions:

b) 3+2, 6–3, 4+5, 9–2, 4+1, 7 – 2, 10 – 1, 6+1, 3+4. (By dividing this set of expressions into groups, students can focus not only on the sign of the arithmetic operation, but also on the result.)

When starting new tasks, children usually first focus on the signs that occurred when performing previous tasks. In this case, it is useful to specify the number of split groups. For example, for the expressions: 3+2, 4+1, 6+1, 3+4, 5+2, you can offer a task in the following formulation: “Divide the expressions into three groups according to some criterion.” Students, naturally, first focus on the sign of the arithmetic operation, but then the division into three groups does not work. They begin to focus on results, but they also end up with only two Groups. During the search, it turns out that it is possible to divide into three groups, focusing on the value of the second term (2, 1, 4).

A computational technique can also serve as a basis for dividing expressions into groups. For this purpose, you can use a task of this type: “On what basis can these expressions be divided into two groups: 57+4, 23+4, 36+2, 75+2, 68+4, 52+7.76+7.44 +3.88+6, 82+6?”

If students cannot see the necessary basis for classification, then the teacher helps them as follows: “In one group I will write the following expression: 57 + 4,” he says, “in another: 23 + 4. In which group will you write the expression 36+9?” If in this case the children find it difficult, then the teacher can give them a reason: “What computational technique do you use to find the meaning of each expression?”

Classification tasks can be used not only for productive consolidation of knowledge, skills and abilities, but also when introducing students to new concepts. For example, to define the concept of “rectangle” to a set of geometric shapes located on a flannelgraph, you can offer the following sequence of tasks and questions:

Remove the “extra” figure. (Children remove the triangle and actually divide the set of shapes into two groups, focusing on the number of sides and angles in each shape.)

How are all the other figures similar? (They have 4 angles and 4 sides) V What can you call all these shapes? (Quadrangles.)

Show quadrilaterals with one right angle (6 and 5). (To test their guess, students use a model of a right angle, applying it appropriately to the indicated figure.)

Show quadrilaterals: a) with two right angles (3 and 10);

b) with three right angles (there are none); c) with four right angles (2, 4, 7, 8, 9).

Divide the quadrilaterals into groups according to the number of right angles (1st group - 5 and 6, 2nd group - 3 and 10, 3rd group - 2, 4, 7, 8, 9).

The quadrangles are laid out accordingly on the flannelgraph. The third group includes quadrilaterals in which all angles are right. These are rectangles.

Thus, when teaching mathematics, you can use classification tasks of various types:

1. Preparatory tasks. These include: “Remove (name) the “extra” object”, “Draw objects of the same color (shape, size)”, “Give a name to the group of objects.” This also includes tasks for developing attention and observation:

“What item was removed?” and “What has changed?”

2. Tasks in which the teacher indicates the basis of the classification.

3. Tasks in which children themselves identify the basis of classification.

Activity 87. Create different types of classification tasks that you could give students when learning about geometry, division with a remainder, computational techniques for oral multiplication and division within 100, and also when introducing the square.

3.5. Technique of analogy

The concept of “analogous” translated from Greek means “similar”, “corresponding”, the concept of analogy is similarity in any respect between objects, phenomena, concepts, methods of action.

In the process of teaching mathematics, the teacher quite often tells the children: “Do it by analogy” or “This is a similar task.” Typically, such instructions are given with the aim of securing certain actions (operations). For example, after considering the properties of multiplying a sum by a number, various expressions are proposed:

(3+5) 2, (5+7) 3, (9+2) *4, etc., with which actions similar to this example are performed.

But another option is also possible when, using an analogy, students find new ways of activity and test their guess. In this case, they themselves must see the similarity between objects in some respects and independently make a guess about the similarity in other respects, i.e., draw a conclusion by analogy. But in order for students to be able to make a “guess,” it is necessary to organize their activities in a certain way. For example, students learned an algorithm for written addition of two-digit numbers. Moving on to the written addition of three-digit numbers, the teacher asks them to find the meanings of the expressions: 74+35, 68+13, 54+29, etc. After this, he asks: “Who can guess how to add these numbers: 254+129?” It turns out that in the cases considered, two numbers were added, the same is proposed in the new case. When adding two-digit numbers, they were written one under the other, focusing on their bit composition, and added bit by bit. A guess arises - it’s probably possible to add three-digit numbers in the same way. The teacher can give a conclusion about the correctness of the guess or invite the children to compare the actions performed with the model.

Inference by analogy can also be used when moving on to written addition and subtraction of multi-digit numbers, comparing it with addition and subtraction of three-digit numbers.

Inference by analogy can be used when studying the properties of arithmetic operations. In particular, the commutative property of multiplication. For this purpose, students are first asked to find the meanings of the expressions:

6+3 7+4 8+4 3+6 4+7 4+8

– What property did you use when completing the task? (Commutative property of addition).

– Think about it: how do you determine whether the commutative property holds for multiplication?

By analogy, students write down pairs of products and find the value of each, replacing the product with the sum.

To make a correct inference by analogy, it is necessary to identify the essential features of objects, otherwise the conclusion may turn out to be incorrect. For example, some students try to apply the method of multiplying a number by a sum when multiplying a number by a product. This suggests that the essential property of this expression - multiplication by a sum - was outside their field of vision.

When developing in younger schoolchildren the ability to make inferences by analogy, it is necessary to keep in mind the following:

Analogy is based on comparison, so the success of its application depends on how well students are able to identify the characteristics of objects and establish the similarities and differences between them.

To use an analogy, you must have two objects, one of which is known, the second is compared with it according to some characteristics. Hence, the use of analogy helps to repeat what has been learned and systematize knowledge and skills.

To orient schoolchildren to the use of analogy, it is necessary to explain to them the essence of this technique in an accessible form, drawing their attention to the fact that in mathematics a new method of action can often be discovered by guessing, remembering and analyzing a known method of action and a given new task.

For correct actions, the characteristics of objects that are significant in a given situation are compared by analogy. Otherwise the output may be incorrect.

Task 88. Give examples of inferences by analogy that can be used when studying algorithms for written multiplication and division.

3.6. Generalization technique

Identification of essential features of mathematical objects, their properties and relationships is the main characteristic of such a method of mental action as generalization.

It is necessary to distinguish between the result and the process of generalization. The result is recorded in concepts, judgments, rules. The process of generalization can be organized in different ways. Depending on this, they speak of two types of generalization – theoretical and empirical.

In elementary mathematics courses, the empirical type is most often used, in which generalization of knowledge is the result of inductive reasoning (inferences).

Translated into Russian, “induction” means “guidance,” therefore, using inductive reasoning, students can independently “discover” mathematical properties and methods of action (rules), which are strictly proven in mathematics.

To obtain a correct generalization inductively it is necessary:

1) think over the selection of mathematical objects and the sequence of questions for targeted observation and comparison;

2) consider as many private objects as possible in which the pattern that students should notice is repeated;

3) vary the types of particular objects, i.e. use subject situations, diagrams, tables, expressions, reflecting the same pattern in each type of object;

4) help children verbally formulate their observations by asking leading questions, clarifying and correcting the formulations that they offer.

Let's look at a specific example of how the above recommendations can be implemented. In order to lead students to the formulation of the commutative property of multiplication, the teacher offers them the following tasks:

Look at the picture and try to quickly calculate how many windows there are in the house.

Children can suggest the following methods: 3+3+3+3, 4+4+4 or 3*4=12; 4*3=12.

The teacher suggests comparing the obtained equalities, i.e., identifying their similarities and differences. It is noted that both products are the same, and the factors are rearranged.

Students perform a similar task with a rectangle, which is divided into squares. The result is 9*3=27; 3*9=27 and verbally describe the similarities and differences that exist between the written equalities.

Students are asked to work independently: find the meanings of the following expressions, replacing multiplication with addition:

3*2 4*2 3*6 4*5 5*3 8*4 2*3 2*4 6*3 5*4 3*5 4*8

It turns out how the equalities in each column are similar and different. Answers can be: “The factors are the same, they are rearranged,” “The products are the same,” or “The factors are the same, they are rearranged, the products are the same.”

The teacher helps formulate the property with a guiding question: “If the factors are rearranged, what can be said about the product?”

Conclusion: “If the factors are rearranged, the product will not change” or “The value of the product will not change if the factors are rearranged.”

Task 89. Select a sequence of tasks that can be used to perform inductive inferences when studying:

a) the rules “If the product of two numbers is divided by one factor, we get another”:

b) the commutative properties of addition;

c) the principle of the formation of a natural series of numbers (if we add one to a number, we get the next number when counting; if we subtract 1, we get the previous number);

d) relationships between the dividend, divisor and quotient;

e) conclusions: “the sum of two consecutive numbers is an odd number”; “if you subtract the previous one from the subsequent number, you get I”; “the product of two consecutive numbers is divided by 2”; “If you add to any number and then subtract the same number from it, you get the original number.”

Describe the work with these tasks, taking into account the methodological requirements for the use of inductive reasoning when learning new material.

When developing in younger schoolchildren the ability to generalize observed facts inductively, it is useful to offer tasks in which they may make incorrect generalizations.

Let's look at a few examples:

Compare the expressions, find the commonality in the resulting inequalities and

draw the appropriate conclusions:

2+3 ...2*3 4+5...4*5 3+4...3*4 5+6...5*6

Comparing these expressions and noting the patterns: the sum is written on the left, the product of two consecutive numbers on the right; the sum is always less than the product, most children conclude: “the sum of two consecutive numbers is always less than the product.” But the generalization expressed is erroneous, since the following cases are not taken into account:

0+1 ...0*1

1+2... 1*2

You can try to make a correct generalization, which will take into account certain conditions: “the sum of two consecutive numbers, starting with the number 2, is always less than the product of these same numbers.”

Find the amount. Compare it with each term. Draw the appropriate conclusion.

Term

Based on the analysis of the considered special cases, students come to the conclusion that: “the sum is always greater than each of the terms.” But it can be refuted, since: 1+0=1, 2+0=2. In these cases, the sum is equal to one of the terms.

V Check whether each term is divisible by 2 and draw a conclusion.

(2+4):2=3 (4+4):2=4 (6+2):2=4 (6+8):2=7 (8+10):2=9

Analyzing the proposed special cases, children can come to the conclusion that: “if the sum of numbers is divisible by 2, then each term of this sum is divisible by 2.” But this conclusion is erroneous, since it can be refuted: (1+3):2. Here the sum is divided by 2, each term is not divisible.

Task 90. ​​Using the content of the elementary mathematics course, come up with tasks in which students can make incorrect inductive conclusions.

Most psychologists, teachers and methodologists believe that empirical generalization, which is based on the action of comparison, is most accessible to younger schoolchildren. This, in fact, determines the construction of a mathematics course in primary school.

By comparing mathematical objects or methods of action, the child identifies their external common properties, which can become the content of the concept. However, focusing on the external, perceptible properties of the compared mathematical objects does not always allow one to reveal the essence of the concept being studied or to assimilate the general method of action. When making empirical generalizations, students often focus on unimportant properties of objects and on specific situations. This has a negative impact on the formation of concepts and general methods of action. For example, when forming the concept of “more by,” the teacher usually offers a series of specific situations that differ from each other only in numerical characteristics. In practice, it looks like this: children are asked to put three red circles in a row, put the same number of blue ones under them, then find out how to make the number of circles in the bottom row increase by 2 (add 2 circles). Then the teacher suggests putting 5 (4,6,7 ...) circles in the first row, and 3 (2,5,4 ...) more in the second row. It is assumed that as a result of completing such tasks, the child will form the concept of “more by”, which will find its expression in the method of action: “take the same amount and more...”. But, as practice shows, the focus of students’ attention in this case, first of all, remains various numerical characteristics, and not the general method of action itself. Indeed, having completed the first task, the student can only draw a conclusion about how to “do more by 2” by completing the following tasks - “how to do more by 3 (by 4, by 5)”, etc. As a result, the generalized verbal the formulation of the method of action: “you need to take the same amount and more” is given by the teacher, and most children learn the concept of “more by” only as a result of performing monotonous training exercises. Therefore, they are able to perform certain reasoning only within a given specific situation and on a limited range of numbers.

Unlike empirical, theoretical generalization is carried out by analyzing data about any one object or situation in order to identify significant internal connections. These connections are immediately fixed abstractly (theoretically - with the help of words, signs, diagrams) and become the basis on which private (concrete) actions are subsequently carried out.

A necessary condition for the formation of the ability for theoretical generalization in younger schoolchildren is the focus of education on the formation of general methods of activity. To fulfill this condition, it is necessary to think through such actions with mathematical objects, as a result of which children will be able to “discover” the essential properties of the concepts being studied and the general ways of acting with them.

The development of this issue at the methodological level presents a certain difficulty. At present, this is one of the most pressing problems of primary education, the solution of which is associated both with a change in content and with a change in the organization of educational activities of primary schoolchildren, aimed at mastering it.

Significant changes have been made to the course of elementary mathematics (V.V. Davydov), the goal of which is to develop children's ability to make theoretical generalizations. They relate to both its content and ways of organizing activities. The basis of theoretical generalizations in this course is substantive actions with quantities (length, volume), as well as various techniques for modeling these actions using geometric figures and symbols. This creates certain conditions for making theoretical generalizations. Let's consider a specific situation that is associated with the formation of the concept “more on.” Students are offered two jars. One (first) is filled with water, the other (second) is empty. The teacher suggests finding a way to solve the following problem: how to make sure that the second jar of water contains this glass (shows a glass of water) more than the first? As a result of discussing various proposals, the conclusion is drawn: you need to pour water from the first jar into the second, that is, pour into the second the same amount of water as was poured into the first jar, and then pour another glass of water into the second. The created situation allows the children to find the necessary method of action themselves, and the teacher to focus on the essential feature of the concept “more by,” i.e., to direct students to master the general method of action: “the same and more.”

The use of quantities to develop generalized methods of action in schoolchildren is one of the possible options for constructing an initial mathematics course. But the same problem can be solved by performing various actions and with many objects. Examples of such situations are reflected in the articles of G. G. Mikulina .

She advises using a situation with multiple objects to form the concept of “more on”: children are offered a pack of red cards. You need to fold a pack of green cards so that it contains this much more (a pack of blue cards is shown) than a pack of red cards. Condition: cards cannot be counted.

Using the method of establishing a one-to-one correspondence, students lay out as many cards in the green pack as there are in the red pack, and add another third pack (of blue cards) to it.

Along with empirical and theoretical generalizations, generalizations-agreements take place in a mathematics course. Examples of such generalizations are the rules of multiplication by 1 and by 0, which are valid for any number. They are usually accompanied by explanations:

“in mathematics it is agreed...”, “in mathematics it is generally accepted...”.

Task 91. Using the content of the elementary mathematics course, come up with situations for theoretical and empirical generalization when studying any concept, property or method of action.

3.7. Ways to substantiate the truth of judgments

An indispensable condition for developmental education is the formation in students of the ability to substantiate (prove) the judgments that they express. In practice, this ability is usually associated with the ability to reason and prove one’s point of view.

Judgments can be single: in them something is affirmed or denied regarding one object. For example: “The number 12 is even; square ABCD has no sharp corners; the equation 23 – x = 30 has no solution (within the primary grades), etc.”

In addition to individual judgments, a distinction is made between private and general judgments. In particulars, something is affirmed or denied regarding a certain set of objects from a given class or regarding a certain subset of a given set of objects. For example: “The equation x – 7 = 10 is solved based on the relationship between the minuend, the subtrahend, and the difference.” In this judgment we are talking about an equation of a particular type, which is a subset of the set of all equations studied in primary grades.

In general judgments, something is affirmed or denied regarding all objects of a given set. For example:

"In a rectangle, opposite sides are equal." Here we are talking about anyone, i.e. about all rectangles. Therefore, the judgment is general, although the word “all” is absent in this sentence. Any equation in the primary grades is solved on the basis of the relationship between the results and the components of arithmetic operations. This is also a general proposition, since it covers all kinds of equations found in elementary school mathematics courses.

Sentences expressing judgments can be different in form: affirmative, negative, conditional (for example: “if a number ends in zero, then it is divisible by 10”).

As is known, in mathematics, all propositions, with the exception of the initial ones, as a rule, are proven deductively. The essence of deductive reasoning comes down to the fact that, on the basis of some general judgment about objects of a given class and some individual judgment about a given object, a new individual judgment about the same object is expressed. It is customary to call a general judgment a general premise, the first individual judgment a particular premise, and a new individual judgment a conclusion. Let, for example, you need to solve the equation: 7*x=14. To find an unknown factor, the rule is used: “If the value of the product is divided by one factor (known), we get another (the value of the unknown factor).”

This rule (general judgment) is a general premise. In this equation, the product is 14, the known factor is 7. This is a particular premise.

Conclusion: “you need to divide 14 by 7, we get 2.” The peculiarity of deductive reasoning in the elementary grades is that they are used in an implicit form, i.e., the general and particular premises are in most cases omitted (not spoken out), students immediately begin an action that corresponds to the conclusion.

Therefore, in fact, it seems that deductive reasoning is absent in the primary school mathematics course.

To consciously carry out deductive inferences, a lot of preparatory work is required, aimed at mastering the conclusion, patterns, properties in general, associated with the development of students’ mathematical speech. For example, quite a long work on mastering the principle of constructing a natural series of numbers allows students to master the rule:

“If you add 1 to any number, you get the next number; If we subtract 1 from any number, we get the number preceding it.”

By compiling tables P+1 and P – 1, the student actually uses this rule as a general premise, thereby performing deductive reasoning. An example of deductive reasoning in primary mathematics teaching is the following reasoning:

"4

Deductive reasoning occurs in elementary mathematics and in calculating the meaning of expressions. The rules for the order of performing actions in expressions act as a general premise; as a particular premise, a specific numerical expression is used, when finding the value of which students are guided by the rule for the order of performing actions.

An analysis of school practice allows us to conclude that all methodological possibilities are not always used to develop students’ reasoning skills. For example, when performing a task:

Compare expressions by putting a sign<.>or = to get the correct entry:

6+3 ... 6+2 6+4 ... 4+6

Students prefer to replace reasoning with calculations:

"6+2 . She offered the children two sheets of paper, on one of which general premises were written, on the other – private ones. It is necessary to establish which general premise each particular one corresponds to. Students are given instructions: “You must complete each task on sheet 2 without resorting to calculations, but only using one of the rules written on sheet 1.”

Task 92. Following the instructions above, complete this task.

Sheet 1

1. If the minuend is increased by several units without changing the subtrahend, then the difference will increase by the same number of units.

2. If the divisor is reduced several times without changing the dividend, then the quotient will increase by the same amount.

3. If one of the terms is increased by several units without changing the other, then the sum will increase by the same number of units.

4. If each term is divisible by a given number, then the sum will also be divided by this number.

5. If we subtract the number preceding it from a given number, we get...

Sheet 2

The tasks are arranged in a different sequence than the parcels.

1. Find the difference between 84 – 84, 32 – 31, 54 – 53.

2. Name the sums that are divisible by 3: 9+27, 6+9, 5+18, 12+24, 3+4, "+6.

3. Compare expressions and put signs<.>or = :

125–87 ... 127–87 246–93 ... 249–93 584–121... 588– 121

4. Compare the expressions and put the signs or =:

304:8 ... 3044 243:9 ... 243:3 1088:4 . . 1088:2

5. How to quickly find the sum in each column:

9999 12 15 12 16 30 30 32 32 40 40 40 40 Answer: 91.

Thus, deductive reasoning can be one of the ways to substantiate the truth of judgments in the initial mathematics course. Considering that they are not available to all primary schoolchildren, other methods of substantiating the truth of judgments are used in the primary grades, which in a strict sense cannot be classified as evidence. These include experimentation, calculations and measurements.

An experiment usually involves the use of visualization and objective actions. For example, a child can justify the judgment 7 > 6 by placing 7 circles in one row, with 6 underneath it. Having established a one-to-one correspondence between the circles of the first and second row, he actually substantiates his judgment (in the first row there is one circle without a pair, “an extra ", which means 7>6). The child can turn to objective actions to justify the truth of the result obtained when adding, subtracting, multiplying and dividing, when answering the questions: “How much is one number more (less) than another?”, “How many times is one number more (less) than another ?. Subject actions can be replaced by graphic drawings and drawings. For example, to justify the result of division 7:3=2 (remaining 1), he can use the following figure:

To develop in students the ability to substantiate their judgments, it is useful to offer them tasks to choose a method of action (both methods can be: a) correct, b) incorrect, c) one is correct, the other is incorrect). In this case, each proposed way to complete a task can be considered as a judgment, to justify which students must use various methods of evidence.

For example, when studying the topic “Area Units,” students are offered the task (M2I):

How many times is the area of ​​rectangle ABCD greater than rectangle KMEO? Write your answer as a numerical equation.

Masha wrote down the following equalities: 15:3=5, 30:6=5.

Misha – this is the equality: 60:12=5.

Which one is right? How did Misha and Masha reason?

To substantiate the judgments expressed by Misha and Masha, students can use both the method of deductive reasoning, where the rule of multiple comparison of numbers acts as a general premise, and practical one. In this case, they rely on the given figure.

When proposing a way to solve a problem, students also make judgments, using the mathematical content given in the plot of the problem to prove them. The method of selecting ready-made judgments activates this activity. Examples of tasks include:

On the first day, tourists walked 18 km; on the second day, moving at the same speed, they walked 27 km. At what speed did the tourists walk if they spent 9 hours on the entire journey?

Misha wrote down the solution to the problem as follows:

1) 18:9=2 (km/h)

2) 27:9=3 (km/h)

3) 2+3=5 (km/h) Masha – like this:

1) 18+27=45 (km)

2) 45:9=5 (km/h) Which one is right: Misha or Masha?

How many potatoes were collected from 10 bushes, if from three bushes there were 7 potatoes, from four bushes 9, from six to 8, and from seven bushes 4 potatoes? Masha solved the problem like this:

1)7*3=21 (k.)

2) 4*7=28 (k.)

3) 21+28=49 (k.) Answer: 49 potatoes were collected from 10 bushes. And Misha solved the problem like this:

1)9 4=36 (k.)

2) 8*6=48 (k.)

3) 36+48=84 (k.) Answer: 84 potatoes were collected from 10 bushes. Which one is right?

The process of completing any task should always represent a chain of judgments (general, particular, individual), to justify the truth of which students use various methods.

Let's show this using an example of tasks:

V Insert the numbers into the “boxes” to get the correct equations:

P: 6 = 27054 P:7 = 4083 (rest. 4)

Students express a general judgment: “if we multiply the value of the quotient by the divisor, we get the dividend.” Particular judgment: “the value of the quotient is 27054, the divisor is b.” Conclusion:

"27054*6".

Now the written multiplication algorithm acts as a general premise, the result is found: 162324. The judgment is expressed: 162324: 6 = 27054.

The truth of this judgment can be verified by performing division with a corner or using a calculator.

Do the same with the second entry.

Make up correct equalities using the numbers: 6, 7, 8, 48, 56.

Students make judgments:

6*8=48 (justification – calculations) 56 – 48=8 (justification – calculations)

8*6=48 (to substantiate the judgment, you can use the general premise: “the value of the product will not change by rearranging the factors”).

48:8 = 6 (a general premise is also possible, etc.)" Thus, in most cases, to justify the truth of judgments in the initial course of mathematics, students turn to calculations and deductive reasoning. Thus, justifying the result when solving an example on the order of action, they use a general premise in the form of a rule for the order of actions, then perform calculations.

Measurement as a way to substantiate the truth of judgments is usually used in the study of quantities and geometric material. For example, children can justify the judgments: “the blue segment is longer than the red one,” “the sides of the quadrilateral are equal,” “one side of the rectangle is larger than the other” by measurement.

Task 93. Describe ways to justify the truth of judgments. expressed by students when completing the following tasks. When studying what questions in a primary school mathematics course it is advisable to offer these tasks 9

9*7+9+5 8*6+8+3 7*9+9+5 8*7+3 9*8+5 7*8+3

Is it possible to say that the meanings of the expressions in each column are the same:

12*5 16*4 (8+4)*5 (8+8)*4 (7+5)*5 (9+7)*4 (10+2)*5 (10+6)*4

Insert signs or = to make the correct entries:

(14+8)*3 ... 14*3+8*3 (27+8)*6 ...27*6+8 (36+4)*18 ...40*18 .

What action signs need to be inserted into the “windows” to get the correct equalities

8*8=8P7P8 8*3=8P4P8 8*6=6P8P0 8*5=8P0P32

Is it possible to say that the meanings of the expressions in each column are the same:

8*(4*6) (9*3)*3 8*24 2*27 (8*4)*6 9*(3*2) 6*32 (2*3)*9

3.8. The relationship between logical and algorithmic thinking of schoolchildren

The ability to consistently, clearly and consistently express one’s thoughts is closely related to the ability to present a complex action in the form of an organized sequence of simple ones. This skill is called algorithmic. It finds its expression in the fact that a person, seeing the final goal, can create an algorithmic prescription or algorithm (if it exists), as a result of which the goal will be achieved.

Drawing up algorithmic instructions (algorithms) is a complex task, so an initial mathematics course does not aim to solve it. But he can and should take upon himself some preparation for achieving it, thereby contributing to the development of logical thinking in schoolchildren.

To do this, starting from the 1st grade, it is necessary, first of all, to teach children to “see” algorithms and to understand the algorithmic essence of the actions that they perform. This work should begin with the simplest algorithms that are accessible and understandable to them. You can create an algorithm for crossing a street with an uncontrolled and controlled intersection, algorithms for using various household appliances, preparing a dish (cooking recipe), presenting the path from home to school, from school to the nearest bus stop, etc. in the form of sequential operations.

The method for preparing a coffee drink is written on the box and is the following algorithm:

1. Pour a glass of hot water into the pan.

2. Take a teaspoon of the drink.

3. Pour (pour) the coffee drink into a pan of water.

4. Heat the contents of the pan to a boil.

5. Let the drink settle.

6. Pour the drink into a glass.

When considering such instructions, the term “algorithm” itself can not be introduced, but we can talk about rules in which points are highlighted indicating certain actions, as a result of which the task is solved.

It should be noted that the term “algorithm” itself can only be used conditionally, since those rules and regulations that are discussed in the primary school mathematics course do not have all the properties that characterize it. Algorithms in elementary grades do not describe the sequence of actions using a specific example in a general form; they do not reflect all the operations that are part of the actions being performed, so their sequence is not strictly defined. For example, the sequence of actions when multiplying numbers ending in zeros by a single-digit number (800*4) is performed as follows:

1. Let's imagine the first factor as a product of a single-digit number and a unit ending in zeros: (8*100) 4;

2. Let’s use the associative property of multiplication:

(8*100)*4 =8 *(100*4);

3. Let's use the commutative property of multiplication:

8*(100*4)=8*(4*100);

4. Let's use the associative property of multiplication:

8*(4*100)=(8*4)*100;

5. Replace the product in brackets with its value:

(8*4)*100 =32*100;

6. When multiplying a number by 1 with zeros, you need to add as many zeros to the number as there are in the second factor:

32*100=3200.

Of course, younger schoolchildren cannot learn the sequence of actions in this form, but by clearly presenting all the operations, the teacher can offer children various exercises, the implementation of which will allow the children to understand the method of activity. For example:

Is it possible, without performing calculations, to say that the values ​​of the expressions in each column are the same:

9*(8*100) 800*7 (9*8)*100 (8*7)*100 (9*100)*8 8*(7*100) 9*100 8*700 72*100 56*100

Explain how you obtained the expression written on the right:

4*6*10=40*6 2*8*10=20*8 8*5*10=8*50 5*7*10=7*50

Is it possible to say that the values ​​of the products in each pair are the same:

45*10 54*10 32*10 9*50 60*9 8*40

In order for children to understand the algorithmic essence of the actions they perform, it is necessary to reformulate these mathematical tasks in the form of a specific program.

For example, the task “find 5 numbers, the first of which is 3, each next one is 2 more than the previous one” can be represented as an algorithmic prescription like this:

1. Write down the number 3.

2. Increase it by 2.

3. Increase the result by 2.

4. Repeat operation 3 until you write down 5 numbers. The verbal algorithmic prescription can be replaced with a schematic one:

This will allow students to more clearly imagine each operation and the sequence in which they are performed.

Task 94. Formulate the following mathematical tasks in the form of algorithmic instructions and present them in the form of a diagram

actions:

a) write 4 numbers, the first of which is 1, each next

2 times more than the previous one;

b) write 4 numbers, the first of which is 0, the second is greater than the first by 1, the third is greater than the second by 2, the fourth is greater than the third by 3;

c) write 6 numbers: if the first is 9, the second is 1, and each next one is equal to the sum of the two previous ones.

Along with verbal and schematic instructions, you can specify the algorithm in the form of a table.

For example, the task: “Write down the numbers from 1 to 6. Increase each:

a) by 2; b) by 3" can be presented in the following table:

+

Thus, algorithmic instructions can be specified verbally, in diagrams and in tables.

By working with specific mathematical objects and generalizations in the form of rules, children master the ability to identify the elementary steps of their actions and determine their sequence.

For example, the rule for checking addition can be formulated as an algorithmic prescription as follows. In order to check addition by subtraction, you need:

1) subtract one of the terms from the sum;

2) compare the result obtained with another term;

3) if the result obtained is equal to another term, then the addition is performed correctly;

4) otherwise look for an error.

Task 95. Make up algorithmic instructions that younger schoolchildren can use when: a) adding single-digit numbers with transition through place value; b) comparison of multi-digit numbers; c) solving equations; d) written multiplication by a single-digit number.

To develop the ability to compose algorithms, you need to teach children: to find a general method of action; highlight the basic, elementary actions that make up the given; plan the sequence of selected actions; write the algorithm correctly.

Let's consider tasks whose goal is to identify a method of action:

The numbers are given (see picture). Make up expressions and find their meanings. How many addition examples can you make? How should one reason in this case so as not to miss a single case?

When completing this task, students realize the need to identify a general method of action. For example, fix the first term 31, add all the numbers in the second column as the second, then fix, for example, the number 41 as the first term and again select all the numbers from the second column, etc. You can fix the second term and go through all the numbers in the first column. It is important that the child understands that by adhering to a certain method of action, he will not miss a single case and will not write down a single case twice.

The hall has three chandeliers and 6 windows. For the holiday, a garland was stretched from each chandelier to each window for decoration. How many garlands did you hang in total? (When solving, you can use a schematic drawing.)

Combinatorial tasks are useful for developing students’ ability to identify a method of action. Their peculiarity is that they have not one, but many solutions, and when executing them, it is necessary to search in a rational sequence. For example:

How many different five-digit numbers can be written using the numbers 55522 (the number 5 can be repeated three times, 2 - twice).

To solve this combinatorial problem, you can use the construction of a “tree”. First, one digit is written down, with which you can start recording the number. The further algorithm of actions comes down to writing down the numbers that can be placed after each digit until we get a five-digit number. Following this algorithm, you need to combine and count how many times the numbers 5 and 2 are repeated.

The result is “branches” with different numbers: 55522, 55252, 55225, 52552, 52525, 52255. Then the number 2 is written out.

We write down the numbers, moving along the “branches”: 22555, 25525, 25552, 25255. Answer: you can write down 10 numbers.

Task 96. Select combinatorial problems that you could offer to first, second and third grade students when studying various concepts in the initial mathematics course.

CHAPTER 4. TRAINING JUNIOR SCHOOL CHILDREN IN PROBLEM SOLVING

4.1. The concept of “problem” in an initial mathematics course

Any mathematical task can be considered as a task by highlighting the condition in it, i.e. the part that contains information about known and unknown values ​​of quantities, the relationships between them, and the requirement (i.e. an indication of what needs to be found) . Let's look at examples of mathematical tasks from a primary school course:

> Put the = signs to get the correct entries: 3 ... 5, 8 ... 4.

The condition of the problem is the numbers 3 and 5, 8 and 4. The requirement is to compare these numbers.

*> Solve the equation: x + 4 = 9.

The condition contains an equation. The requirement is to solve it, that is, substitute such a number for x to obtain a true equality.

Here the condition gives triangles. The requirement is to fold a rectangle.

To fulfill each requirement, a specific method or method of action is used, depending on which different types of mathematical problems are distinguished: construction, proof-